THE UNIT CIRCLE
The set of points at a distance 1 from the origin is a circle of radius 1.
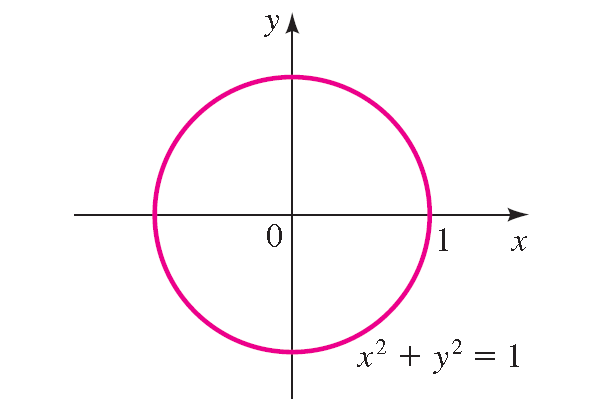
The unit circle is the circle of radius 1 centered at the origin in the xy-plane.
Its equation is
x2 + y2 = 1
A Point on the Unit Circle
Example 1 :
Show that the point P(√3/3, √6/3) is on the unit circle.
Solution :
We have to show that this point satisfies the equation of the unit circle, that is, x2 + y2 = 1.
(√3/3)2 + (√6/3)2 = 3/9 + 6/9
= (3 + 6)/9
= 9/9
= 1
So, P is on the unit circle.
Locating a Point on the Unit Circle
Example 2 :
If the the point P(√3/2, k) is on the unit circle in quadrant IV, find the value of k.
Solution :
Because the point is on the unit circle, we have
(√3/2)2 + k2 = 1
3/4 + k2 = 1
Subtract 3/4 from both sides.
k2 = 1 - 3/4
k2 = 4/4 - 3/4
k2 = (4 - 3)/4
k2 = 1/4
Take square root on both sides.
k = ±1/2
Because the point is in quadrant IV and k is the y-coordinate, the value of k must be negative.
So, k = -1/2.
Terminal Points on the Unit Circle
Suppose t is a real number. Let’s mark off a distance t along the unit circle, starting at the point (1, 0) and moving in a counterclockwise direction, if t is positive, move counter clockwise and if t is negative move clockwise.
In this way we arrive at a point P(x, y) on the unit circle. The point P(x, y) obtained in this way is called the terminal point determined by the real number t.
Terminal Point P(x, y) Determined by t > 0 :
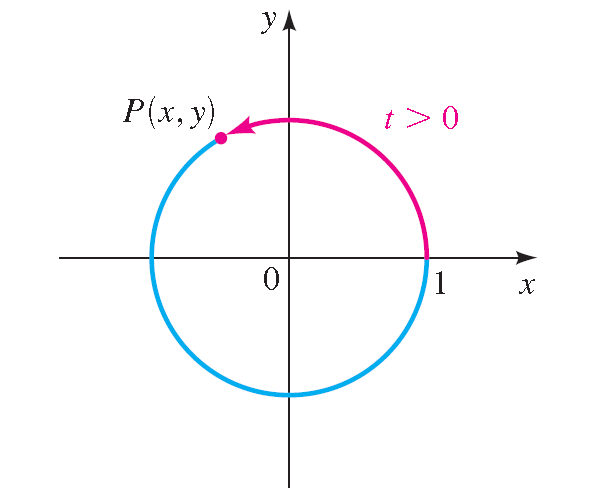
Terminal Point P(x, y) Determined by t < 0 :
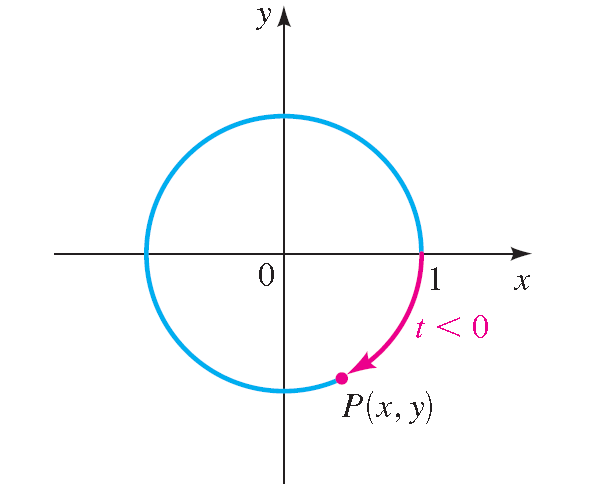
The circumference of the unit circle is
C = 2π(1) = 2π
So, if a point starts at (1, 0) and moves counter clockwise all the way around the unit circle and returns to (1, 0), it travels a distance of 2π. To move halfway around the circle, it travels a distance of (1/2)(2π) = π.
To move a quarter of the distance around the circle, it travels a distance of (1/4)(2π) = π/2. Where does the point end up when it travels these distances along the circle? In the diagram shown below, we see, for example, that when it travels a distance of π starting at (1, 0), its terminal point is (-1, 0).
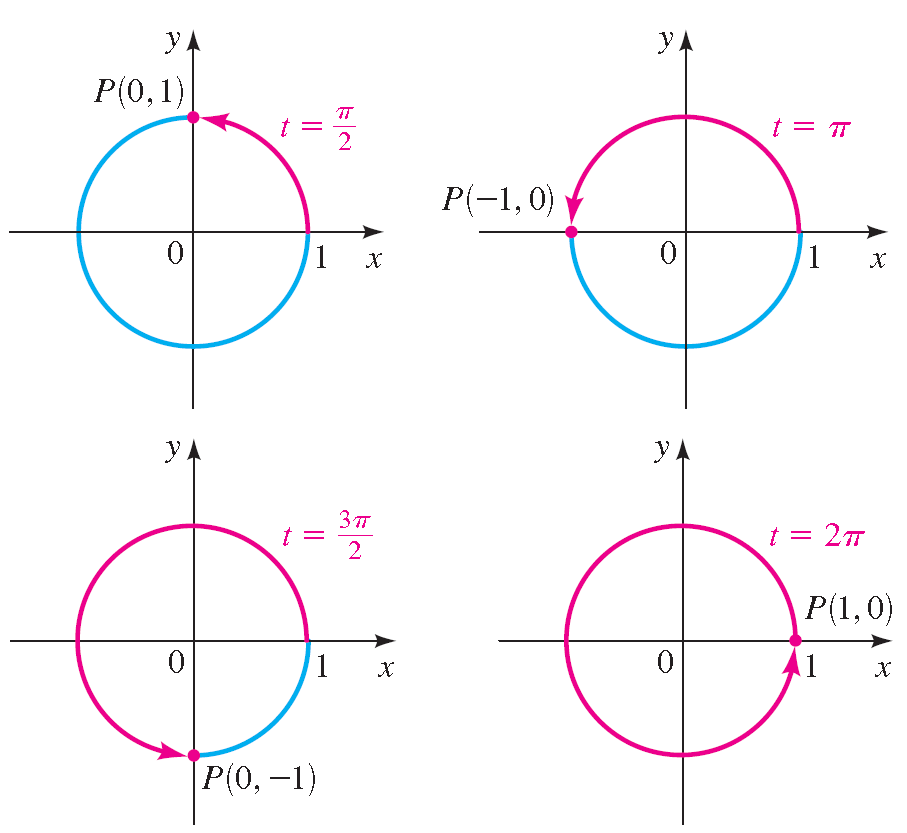
Finding Terminal Points
Find the terminal point on the unit circle determined by each real number t.
Example 3 :
t = 3π
Solution :
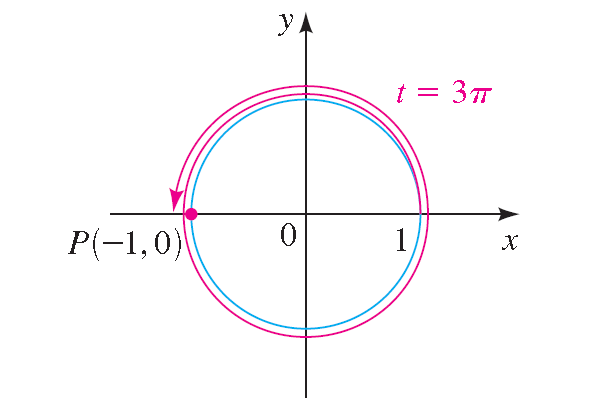
The terminal point determined by 3π is (-1, -0).
Example 4 :
t = -π
Solution :
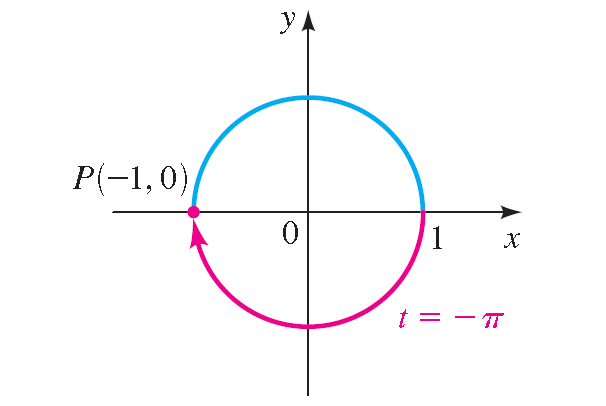
The terminal point determined by -π is (-1, -0).
Example 5 :
t = -π/2
Solution :
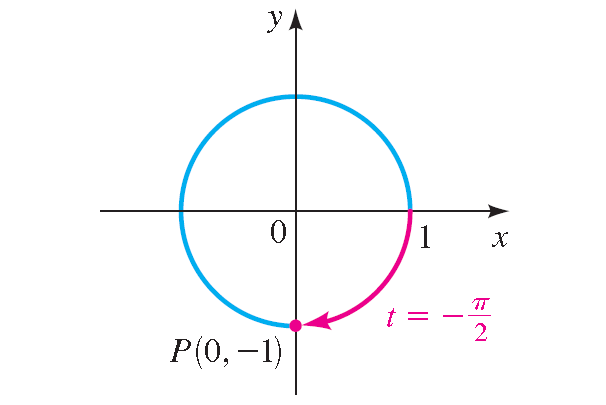
The terminal point determined by -π/2 is (0, -1).
Notice that different values of t can determine the same terminal point.
The terminal point P(x, y) determined by t = π/4 is the same distance from (1, 0) as from (0, 1) along the unit circle.

Since the unit circle is symmetric with respect to the line y = x, it follows that P lies on the line y = x. So P is the point of intersection (in the first quadrant) of the circle x2 + y2 = 1 and the line y = x.
Substituting x for y in the equation of the unit circle.
x2 + x2 = 1
2x2 = 1
x2 = 1/2
k = ± 1/√2
Because P is in the first quadrant, x = 1/√2 and y = x, we have y = 1/√2 also.
Thus the terminal point determined by π/4 is
P(1/√2, 1/√2) = P(√2/2, √2/2)
Similar methods can be used to find the terminal points determined by t = π/6 and t = π/3.
Table :
|
t 0 π/6 π/4 π/3 π/2 |
Terminal point determined by t (1, 0) (√3/2, 1/2) (√2/2, √2/2) (1/2, √3/2) (0, 1) |
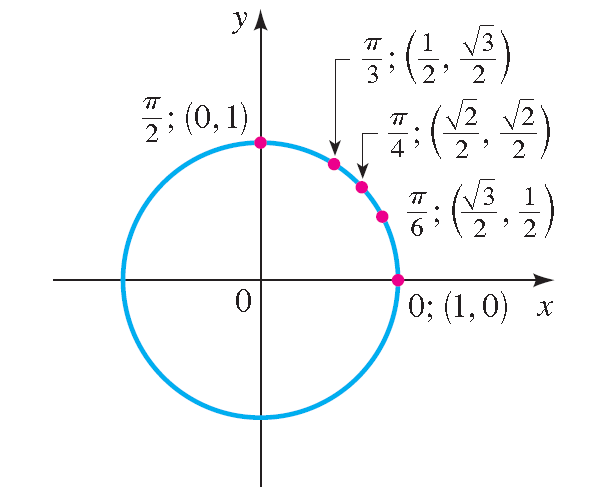
Example 6 :
t = -π/4
Solution :
Let P be the terminal point determined by -π/4, and let Q be the terminal point determined by π/4. In the diagram shown below, we see that the point P has the same coordinates as Q except for sign.
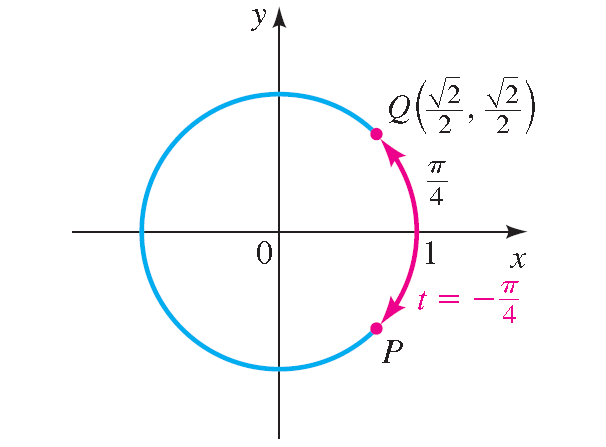
Because P is in quadrant IV, its x-coordinate is positive and its y-coordinate is negative. Thus, the terminal point is
P(√2/2, -√2/2)
Example 7 :
t = 3π/4
Solution :
Let P be the terminal point determined by 3π/4, and let Q be the terminal point determined by π/4. In the diagram shown below, we see that the point P has the same coordinates as Q except for sign.
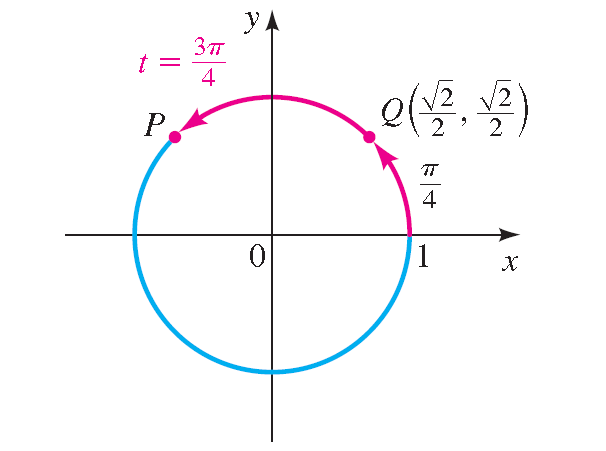
Because P is in quadrant II, its x-coordinate is negative and its y-coordinate is positive. Thus, the terminal point is
P(-√2/2, √2/2)
Example 8 :
t = -5π/6
Solution :
Let P be the terminal point determined by -5π/6, and let Q be the terminal point determined by π/6. In the diagram shown below, we see that the point P has the same coordinates as Q except for sign.
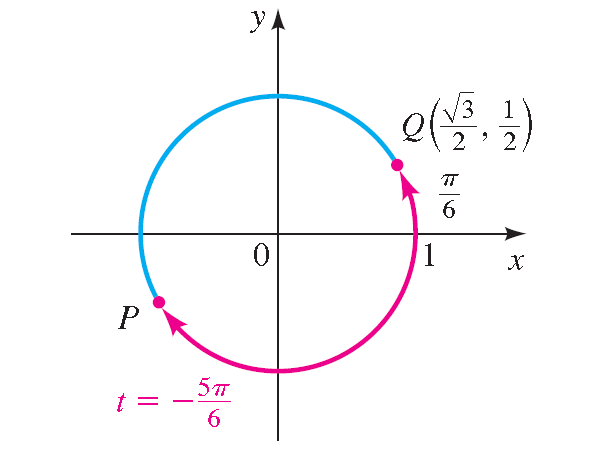
Because P is in quadrant III, its both x-coordinate and y-coordinate are negative. Thus, the terminal point is
P(-√3/2, -1/2)
Reference Number
Let t be a real number. The reference number associated with t is the shortest distance along the unit circle between the terminal point determined by t and the x-axis.
The diagram below shows that to find the reference number t' it’s helpful to know the quadrant in which the terminal point determined by t lies. If the terminal point lies in quadrants I or IV, where x is positive, we find t' by moving along the circle to the positive x-axis. If it lies in quadrants II or III, where x is negative, we find t' by moving along the circle to the negative x-axis.
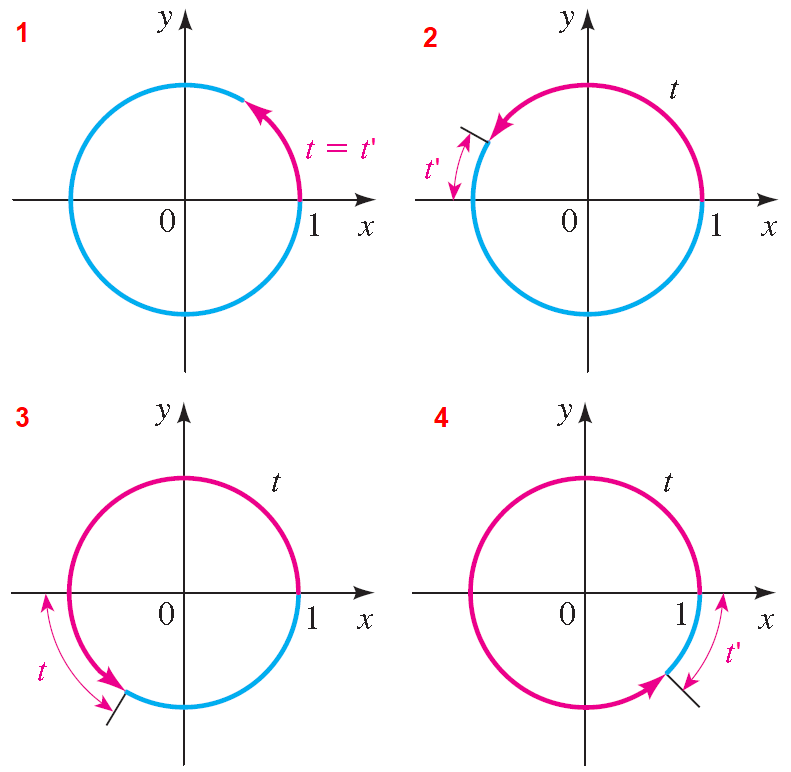
Finding Reference Numbers
Find the reference number for each value of t.
Example 9 :
t = 5π/6
Solution :
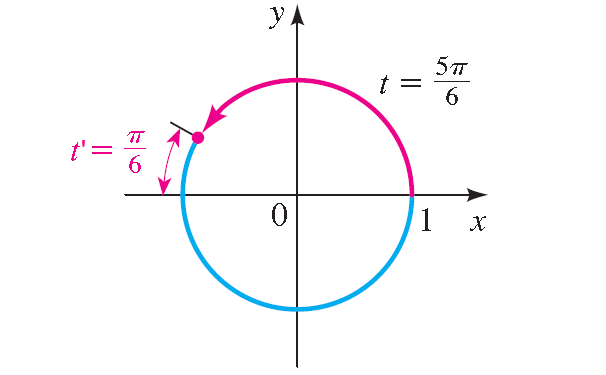
t' = π - 5π/6 = π/6
Example 10 :
t = 7π/4
Solution :
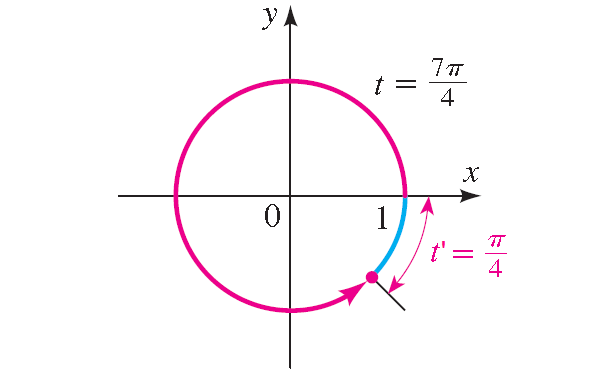
t' = 2π - 7π/4 = π/4
Example 11 :
t = -2π/3
Solution :
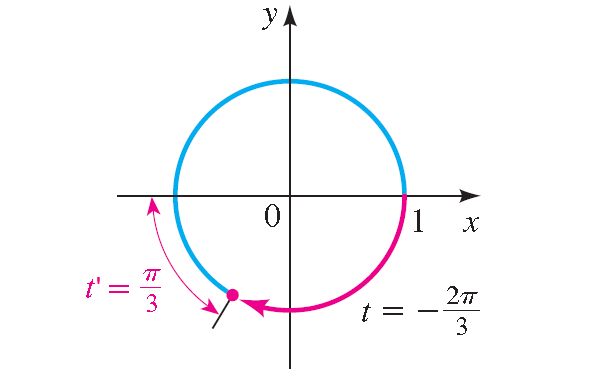
t' = π - 2π/3 = π/3
Example 12 :
t = 5.80
Solution :
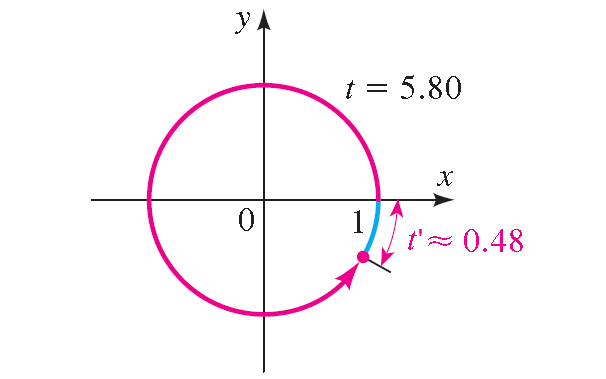
t' = 2π - 5.80 ≈ 0.48
Using Reference Numbers to Find Terminal Points
To find the terminal point P determined by any value of t, you we can use the following steps :
1. Find the reference number.
2. Find the terminal point Q(a, b) determined by t'.
3. The terminal point determined by t is P(±a, ±b), where the signs are chosen according to the quadrant in which this terminal point lies.
Find the terminal point determined by each given real number t.
Example 13 :
t = 5π/6
Solution :
The reference numbers associated with these values of t were found in Example 9.
The reference number is t' = π/6, which determines the terminal point (√3/2, 1/2) from the table shown above.
Because the terminal point determined by t is in quadrant II, its x-coordinate is negative and its y-coordinate is positive. Thus, the desired terminal point is
(-√3/2, 1/2)
Example 14 :
t = 7π/4
Solution :
The reference numbers associated with these values of t were found in Example 10.
The reference number is t' = π/4, which determines the terminal point (√2/2, √2/2) from the table shown above.
Because the terminal point is in quadrant IV, its x-coordinate is positive and its y-coordinate is negative. Thus, the desired terminal point is
(√2/2, -√2/2)
Example 15 :
t = -2π/3
Solution :
The reference numbers associated with these values of t were found in Example 11.
The reference number is t' = π/3, which determines the terminal point (1/2, √3/2) from the table shown above.
Because the terminal point determined by t is in quadrant III, its both x-coordinate and y-coordinate are negative. Thus, the desired terminal point is
(-1/2, -√3/2)
Because the circumference of the unit circle is 2π, the terminal point determined by t is the same as that determined by (t + 2π) or (t - 2π). In general, we can add or subtract 2π any number of times without changing the terminal point determined by t. We can use this observation in the next example to find terminal points for large t.
Finding the Terminal Point for Large t
Example 16 :
Find the terminal point determined by t = 29π/6.
Solution :
Because
t = 29π/6 = 4π + 5π/6
we see that the terminal point of t is the same as that of 5π/6 (that is, we subtract 4π).
So by Example 13 the terminal point is
(-√3/2, 1/2)
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Trigonometry Even and Odd Iidentities
May 05, 24 12:25 AM
Trigonometry Even and Odd Iidentities -
SOHCAHTOA Worksheet
May 03, 24 08:50 PM
SOHCAHTOA Worksheet -
Trigonometry Pythagorean Identities
May 02, 24 11:43 PM
Trigonometry Pythagorean Identities